World's First Quantum Sensing Algorithm Reducing the Effect of Unknown Noise by Quantum Error Mitigation
Realization of High Precision Quantum Sensing without Hardware Improvements

Tokyo −December 16, 2022, −NTT Corporation (TYO:9432, hereinafter referred to as "NTT"), National Institute of Advanced Industrial Science and Technology (hereinafter referred to as "AIST"), and Center for Quantum Information and Quantum Biology, Osaka University (hereinafter referred to as "Osaka University") have devised an algorithm that enables highly accurate sensing using quantum states, quantum sensing¹ using quantum states. In quantum sensing, unknown noise² greatly affects the accuracy, so it is important to reduce its influence. Therefore, we have confirmed for the first time in the world that this algorithm can significantly reduce the effect of unknown noise by utilizing the quantum error mitigation method³ that has been devised for the realization of quantum computers⁴. This enables higher precision quantum sensing without modifying the hardware.

1. Background and History

Sensing technology, which can obtain various types of information such as magnetic fields, electric fields, and temperatures with high accuracy, is becoming increasingly important as one of the fundamental technologies in an increasingly information-oriented society. In particular, there are a wide range of applications in the fields of medicine, biology, and materials engineering, such as nuclear magnetic resonance imaging (MRI), which obtains internal information from magnetic field sensing information, and electroencephalography, which senses brain conditions by detecting electrical activity generated in the brains of humans and animals. The development of sensors that can detect even smaller areas with high accuracy has been long awaited.
　Recently, quantum sensing is expected to surpass the sensitivity and spatial resolution of existing sensors, and research is being conducted worldwide. Quantum sensing has been studied in two main ways. One is the method using superposition states and the other is the method using quantum entangled states⁵.
　Under ideal, noise-free conditions, the entangled-state method is known to be many orders of magnitude more accurate than the superposition method. However, entangled-state methods are susceptible to noise caused by interactions with the external environment and imperfections in the hardware, and reducing the effects of noise is a challenge.

2. Technology Overview

In previous researches on quantum sensing, it has been assumed that information about noise is available in advance (such noise is called known noise hereafter), and hardware improvements have been studied mainly to reduce statistical errors⁶ (Fig. 1(a)).
　In reality, however, not only known noise but also unknown noise has a significant impact on accuracy.
　Therefore, we evaluated the impact of unknown noise on sensing with quantum entangled states by numerical simulations of magnetic field sensing. As a result, we found that systematic errors⁷ occur under unknown noise, which significantly affect the accuracy (Fig. 1(b)).
　It is difficult to deal with such unknown noise using conventional research methods for known noise. By utilizing the virtual distillation method⁸, which is one of the quantum error mitigation methods, we have devised an algorithm that significantly the effect of unknown noise without hardware improvements (Fig. 1(c)).

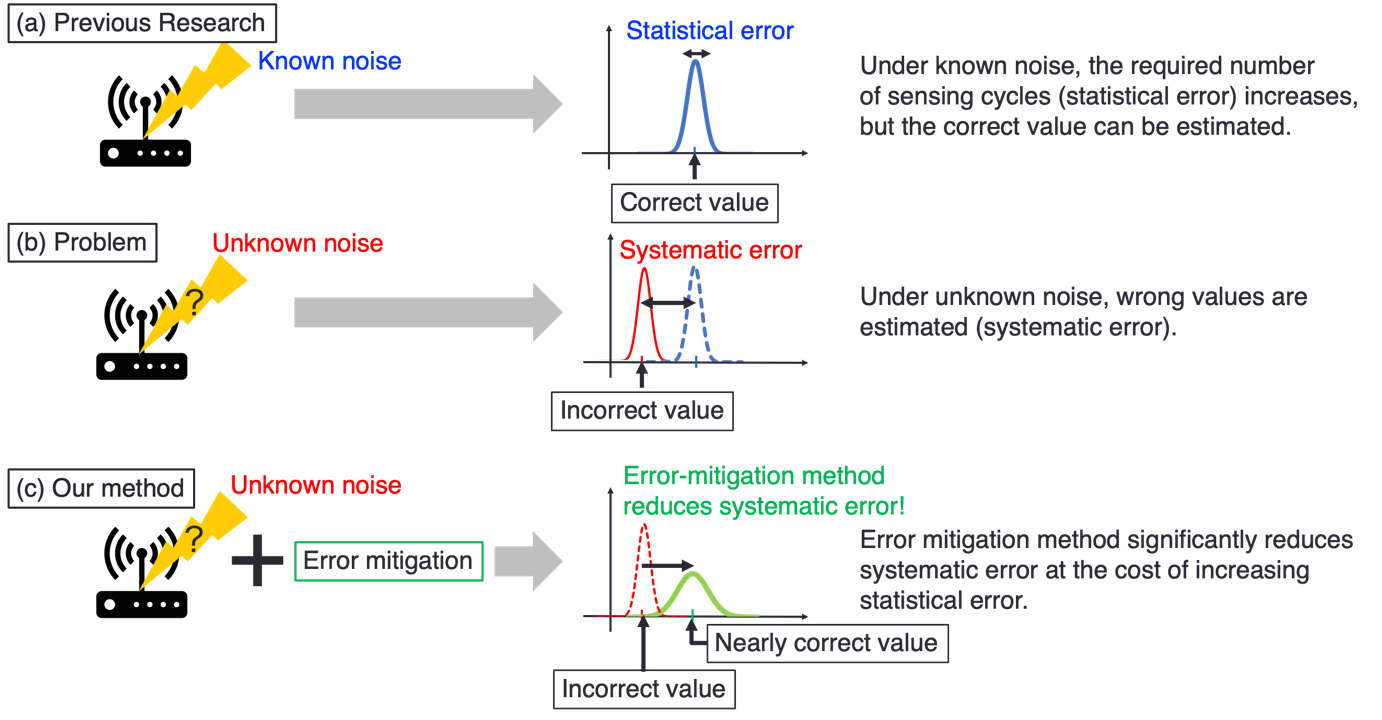Fig. 1: Conceptual diagram of statistical and systematic errors due to known and unknown noise, respectively, and the proposed method.

We have numerically demonstrated that the effect of unknown noise in magnetic field sensing is reduced and the accuracy is improved when the number of qubits comprising the entangled state is large (Fig. 2).
　In this research, NTT proposed the quantum sensing algorithm, analyzed it, and evaluated its performance numerically; AIST proposed the original idea for the algorithm, provided knowledge of quantum sensing, and verified the calculations; and Osaka University verified the analysis calculations of the quantum sensing algorithm.

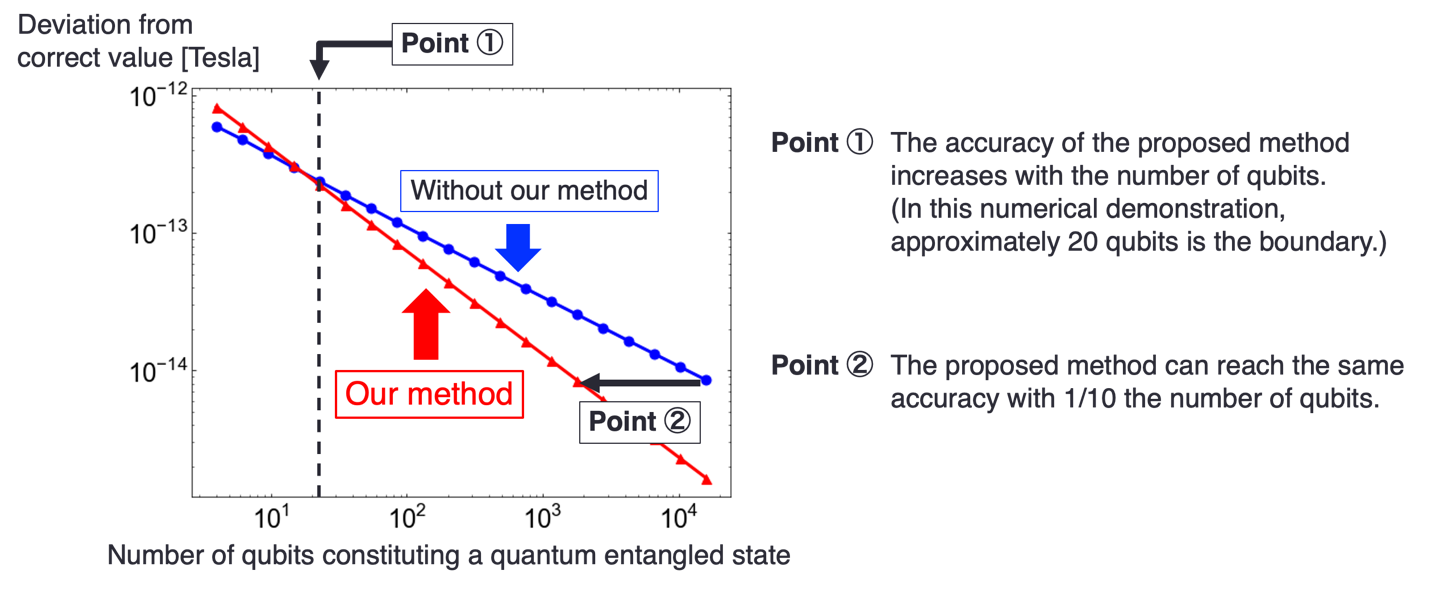Fig. 2: Numerical results of the proposed method applied to magnetic field sensing

3. Future Developments

This research is an important step toward the realization of high-precision quantum sensing using quantum entangled states. Future directions include demonstration experiments of this method and improvement of this method without preparing multiple quantum states. In addition, since this method is expected to be a general method that can be applied to other types of quantum sensing than magnetic field sensing, performance evaluation of this method in other types of quantum sensing is also possible.
　Our algorithm is expected to contribute not only to basic science but also to a wide range of fields where sensing is applied.

4. support for this research

This work was supported by MEXT Q-LEAP Grant No. JPMXS0120319794; JST, PRESTO, Grants No. JPMJPR1919 and JPMJPR2114, Japan; JST COI-NEXT program (JPMJPF2014); JST [Moonshot R&D] Grant No. JPMJMS2061, and JPNP16007, commissioned by the New Energy and Industrial Technology Development Organization (NEDO), Japan.

¹Quantum sensing
Sensing using quantum states. It is used for e.g. detecting magnetic fields, electric fields, temperature, etc.

²Unknown noise
Here, it refers to noise for which no information can be obtained in advance through experiments. Since noise moves stochastically, its exact behavior is basically unknown. However, statistical information such as mean and variance can be known in advance, and this is called known noise. On the other hand, in a real experimental system, there is another type of noise for which even statistical information is not known. This is called unknown noise.

³Error mitigation method
Methods that can reduce errors in quantum computers without burdening the hardware.

⁴Quantum computer
A computer that controls operations based on quantum mechanical principles. Conventional computers are distinguished as "classical computers" because they are based on classical mechanics.

⁵Quantum entanglement
A correlation that cannot be explained by classical mechanics. It is one of the fundamental concepts of quantum mechanics. In quantum sensing, it is known that if a quantum entangled state of a larger number of qubits can be created, more accurate sensing can be achieved.

⁶Statistical Error
A random error resulting from a finite number of observations or measurements. It can be reduced by increasing the number of measurements or the number of samples.

⁷Systematic error
A deviation between the true value and the estimated value. Unlike statistical error, it cannot be reduced by increasing the number of measurements or the number of samples, and thus has a significant impact on accuracy.

⁸Virtual distillation method
A method of error suppression in quantum computers. By "double-checking" multiple quantum states against each other, the effect of noise can be reduced.

This research has been published in the online edition of the American scientific journal Physical Review Letters on December 16, 2022 (Eastern Winter Time).
Journal name: Physical Review Letters (online edition: December 16, 2022)
Title of paper: Error-Mitigated Quantum Metrology via Virtual Purification
Authors: Kaoru Yamamoto^*, Suguru Endo^*, Hideaki Hakoshima^*, Yuichiro Matsuzaki^*, Yuuki Tokunaga^*, and Yuuki Tokunaga^*.

About NTT

NTT believes in resolving social issues through our business operations by applying technology for good. We help clients accelerate growth and innovate for current and new business models. Our services include digital business consulting, technology and managed services for cybersecurity, applications, workplace, cloud, data center and networks all supported by our deep industry expertise and innovation.
As a top 5 global technology and business solutions provider, our diverse teams operate in 88 countries and regions and deliver services to over 190 of them. We serve 85% of Fortune Global 100 companies and thousands of other clients and communities around the world.
For more information on NTT, visit www.global.ntt.